What does parabola mean?
Definitions for parabola
pəˈræb ə ləpar·a·bola
This dictionary definitions page includes all the possible meanings, example usage and translations of the word parabola.
Princeton's WordNet
parabolanoun
a plane curve formed by the intersection of a right circular cone and a plane parallel to an element of the curve
Wiktionary
parabolanoun
The conic section formed by the intersection of a cone with a plane parallel to a tangent plane to the cone; the locus of points equidistant from a fixed point (the focus) and line (the directrix).
parabolanoun
The explicit drawing of a parallel between two essentially dissimilar things, especially with a moral or didactic purpose. A parable.
Etymology: From παραβολή, from παραβάλλω, from παρά + βάλλω.
Samuel Johnson's Dictionary
PARABOLAnoun
The parabola is a conick section, arising from a cone’s being cut by a plane parallel to one of its sides, or parallel to a plane that touches one side of the cone. John Harris
Etymology: Latin.
Had the velocities of the several planets been greater or less than they are now, at the same distances from the sun, they would not have revolved in concentrick circles as they do, but have moved in hyperbola’s or parabola’s, or in ellipses, very excentrick. Richard Bentley, Sermons.
Wikipedia
Parabola
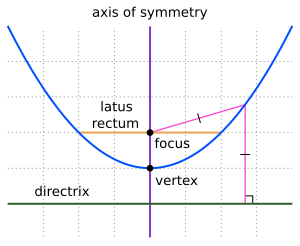
In mathematics, a parabola is a plane curve which is mirror-symmetrical and is approximately U-shaped. It fits several superficially different mathematical descriptions, which can all be proved to define exactly the same curves. One description of a parabola involves a point (the focus) and a line (the directrix). The focus does not lie on the directrix. The parabola is the locus of points in that plane that are equidistant from both the directrix and the focus. Another description of a parabola is as a conic section, created from the intersection of a right circular conical surface and a plane parallel to another plane that is tangential to the conical surface.The line perpendicular to the directrix and passing through the focus (that is, the line that splits the parabola through the middle) is called the "axis of symmetry". The point where the parabola intersects its axis of symmetry is called the "vertex" and is the point where the parabola is most sharply curved. The distance between the vertex and the focus, measured along the axis of symmetry, is the "focal length". The "latus rectum" is the chord of the parabola that is parallel to the directrix and passes through the focus. Parabolas can open up, down, left, right, or in some other arbitrary direction. Any parabola can be repositioned and rescaled to fit exactly on any other parabola—that is, all parabolas are geometrically similar. Parabolas have the property that, if they are made of material that reflects light, then light that travels parallel to the axis of symmetry of a parabola and strikes its concave side is reflected to its focus, regardless of where on the parabola the reflection occurs. Conversely, light that originates from a point source at the focus is reflected into a parallel ("collimated") beam, leaving the parabola parallel to the axis of symmetry. The same effects occur with sound and other waves. This reflective property is the basis of many practical uses of parabolas. The parabola has many important applications, from a parabolic antenna or parabolic microphone to automobile headlight reflectors and the design of ballistic missiles. It is frequently used in physics, engineering, and many other areas.
ChatGPT
parabola
A parabola is a two-dimensional, mirror-symmetrical curve shape, described by a specific form of quadratic equation where any point is at an equal distance from a fixed point (the focus) and a fixed straight line (the directrix). It is the graph of a function that's been squared, typically expressed in the general form y = ax^2 + bx + c. Parabolas can open upwards or downwards (if oriented vertically) or can open left or right (if oriented horizontally).
Webster Dictionary
Parabolanoun
a kind of curve; one of the conic sections formed by the intersection of the surface of a cone with a plane parallel to one of its sides. It is a curve, any point of which is equally distant from a fixed point, called the focus, and a fixed straight line, called the directrix. See Focus
Parabolanoun
one of a group of curves defined by the equation y = axn where n is a positive whole number or a positive fraction. For the cubical parabola n = 3; for the semicubical parabola n = /. See under Cubical, and Semicubical. The parabolas have infinite branches, but no rectilineal asymptotes
Etymology: [NL., fr. Gr. ; -- so called because its axis is parallel to the side of the cone. See Parable, and cf. Parabole.]
Wikidata
Parabola
A parabola is a two-dimensional, mirror-symmetrical curve, which is approximately U-shaped when oriented as shown in the diagram, but which can be in any orientation in its plane. It fits any of several superficially different mathematical descriptions which can all be proved to define curves of exactly the same shape. One description of a parabola involves a point and a line. The focus does not lie on the directrix. The locus of points in that plane that are equidistant from both the directrix and the focus is the parabola. Another description of a parabola is as a conic section, created from the intersection of a right circular conical surface and a plane, which is parallel to a straight line on the conical surface and perpendicular to another plane which includes both the axis of the cone and also the same straight line on its surface. A third description is algebraic. A parabola is a graph of a quadratic function, such as The line perpendicular to the directrix and passing through the focus is called the "axis of symmetry". The point on the axis of symmetry that intersects the parabola is called the "vertex", and it is the point where the curvature is greatest. The distance between the vertex and the focus, measured along the axis of symmetry, is the "focal length". The "latus rectum" is the chord of the parabola which is parallel to the directrix and passes through the focus. Parabolas can open up, down, left, right, or in some other arbitrary direction. Any parabola can be repositioned and rescaled to fit exactly on any other parabola — that is, all parabolas are geometrically similar.²
Chambers 20th Century Dictionary
Parabola
par-ab′o-la, n. (geom.) a curve or conic section, formed by cutting a cone with a plane parallel to its slope (for illustration, see Cone).—adjs. Parabol′ic; Parabol′iform.—n. Parab′oloid, the solid which would be generated by the rotation of a parabola about its principal axis. [Gr. parabolē; cf. Parable.]
The Nuttall Encyclopedia
Parabola
a conic section formed by the intersection of a cone by a plane parallel to one of its sides.
The Standard Electrical Dictionary
Parabola
A curve; one of the conic sections. It is approximately represented by a small arc of a circle, but if extended becomes rapidly deeper than a half circle. If, from a point within called the focus, lines are drawn to the curve and then other lines are drawn from these points parallel to the axis, the angles of incidence will he equal to the angles of reflection as referred to tangents at the points where the lines touch the curve. [Transcriber's note; The general equation of a parabola is A*x^2 + B*x*y + C*y^2 + D*x + E*y + F = 0 such that B^2 = 4*A*C, all of the coefficients are real, and A and C are not zero. A parabola positioned at the origin and symmetrical on the y axis is simplified to y = a*x^2 ]
Dictionary of Nautical Terms
parabola
A geometrical figure formed by the section of a cone when cut by a plane parallel to its side.
Matched Categories
Numerology
Chaldean Numerology
The numerical value of parabola in Chaldean Numerology is: 7
Pythagorean Numerology
The numerical value of parabola in Pythagorean Numerology is: 3
Examples of parabola in a Sentence
Theology I would say can be described as the trajectory of the sacred parabola, where faith ascends into the cosmic heights and descends into the depths of existential contemplation. In this divine curve, each theological reflection becomes a celestial arc, tracing the path of faith's ascent and the graceful descent into the mysteries of the divine.
Popularity rank by frequency of use
References
Translations for parabola
From our Multilingual Translation Dictionary
- ባላAmharic
- القطع المكافئ هندسةArabic
- paràbolaCatalan, Valencian
- parabolaCzech
- ParabelGerman
- παραβολήGreek
- paraboloEsperanto
- parábolaSpanish
- paraabeliFinnish
- paraboleFrench
- parabóilIrish
- પરવલયGujarati
- פרבולהHebrew
- परवलयHindi
- պարաբոլArmenian
- fleygbogiIcelandic
- parabolaItalian
- 放物線Japanese
- 포물선, 抛物線Korean
- പരവലയംMalayalam
- परवलयMarathi
- paraboolDutch
- parabolaPolish
- parábolaPortuguese
- параболаRussian
- paràbola, пара̀болаSerbo-Croatian
- parabelSwedish
- పరావలయంTelugu
- พาราโบลาThai
- parabolTurkish
- parabônVietnamese
- 拋物線Chinese
Get even more translations for parabola »
Translation
Find a translation for the parabola definition in other languages:
Select another language:
- - Select -
- 简体中文 (Chinese - Simplified)
- 繁體中文 (Chinese - Traditional)
- Español (Spanish)
- Esperanto (Esperanto)
- 日本語 (Japanese)
- Português (Portuguese)
- Deutsch (German)
- العربية (Arabic)
- Français (French)
- Русский (Russian)
- ಕನ್ನಡ (Kannada)
- 한국어 (Korean)
- עברית (Hebrew)
- Gaeilge (Irish)
- Українська (Ukrainian)
- اردو (Urdu)
- Magyar (Hungarian)
- मानक हिन्दी (Hindi)
- Indonesia (Indonesian)
- Italiano (Italian)
- தமிழ் (Tamil)
- Türkçe (Turkish)
- తెలుగు (Telugu)
- ภาษาไทย (Thai)
- Tiếng Việt (Vietnamese)
- Čeština (Czech)
- Polski (Polish)
- Bahasa Indonesia (Indonesian)
- Românește (Romanian)
- Nederlands (Dutch)
- Ελληνικά (Greek)
- Latinum (Latin)
- Svenska (Swedish)
- Dansk (Danish)
- Suomi (Finnish)
- فارسی (Persian)
- ייִדיש (Yiddish)
- հայերեն (Armenian)
- Norsk (Norwegian)
- English (English)
Word of the Day
Would you like us to send you a FREE new word definition delivered to your inbox daily?
Citation
Use the citation below to add this definition to your bibliography:
Style:MLAChicagoAPA
"parabola." Definitions.net. STANDS4 LLC, 2024. Web. 14 May 2024. <https://www.definitions.net/definition/parabola>.


Discuss these parabola definitions with the community:
Report Comment
We're doing our best to make sure our content is useful, accurate and safe.
If by any chance you spot an inappropriate comment while navigating through our website please use this form to let us know, and we'll take care of it shortly.
Attachment
You need to be logged in to favorite.
Log In